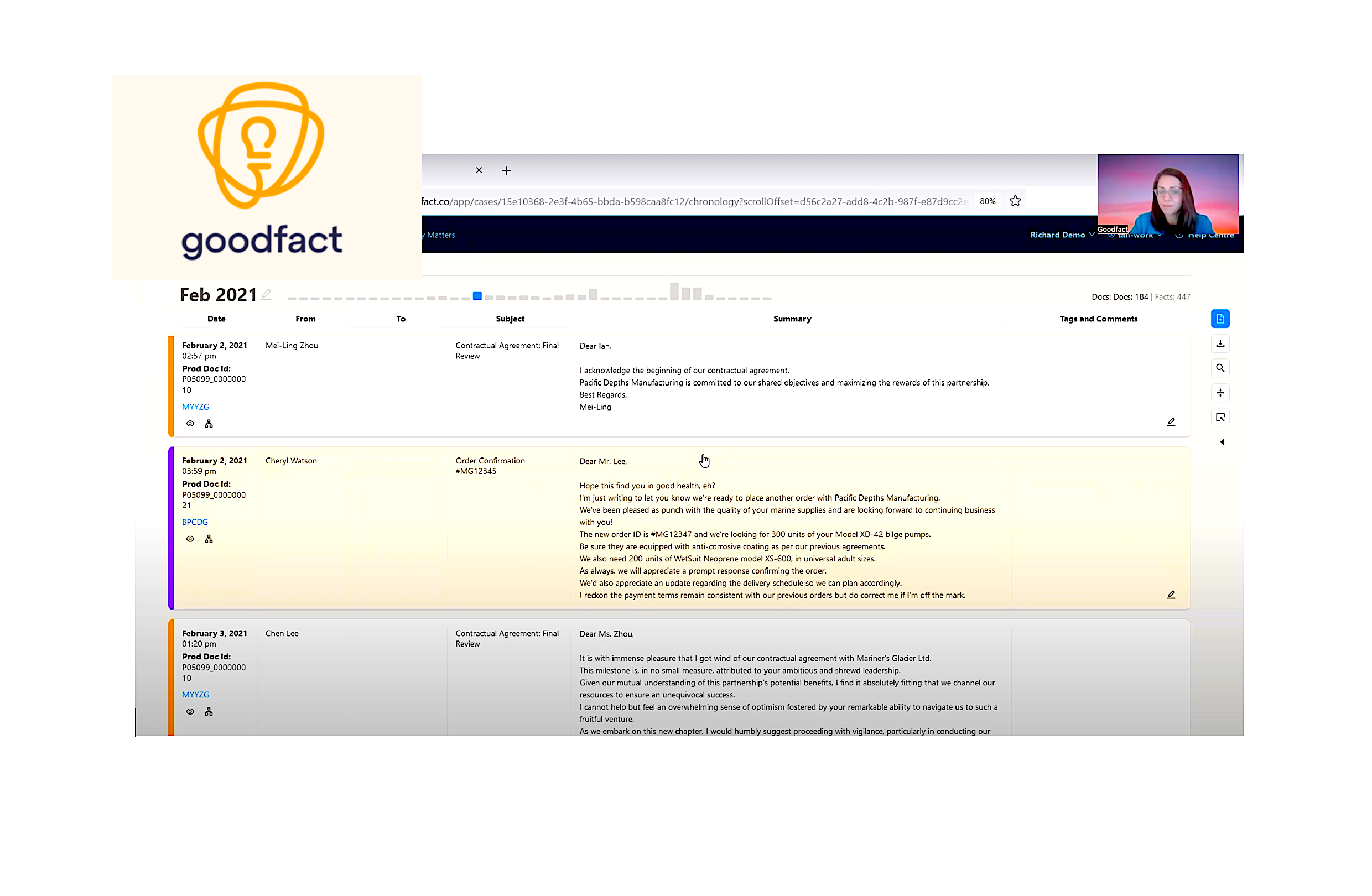
Daily Quantum Learning #2 - Determinism, Probabilism, and Superposition
The last lesson was all about qubits and the potential displayed by them. Now let’s dive into one of the most fascinating and important properties exhibited by qubits: superposition. What is Superposition? This is the quantum mechanical principle where a system or particle can exist in multiple states simultaneously until measured. After measurement the particle or system will collapse into one of the states. How Superposition Challenges Standard Physics: Determinism vs. Probabilism Determinism is the idea in classical mechanics that every object’s properties can be determined and measured definitively, allowing all outcomes to be predicted if all initial conditions are known. Probabilism contradicts this line of thinking by stating that events are described by probabilities rather than certainties. This states that each event has a given chance of occurring and state of an object is not known until it is measured. After measurement all the probabilities collapse into one observed outcome. Until this measurement occurs, the outcomes would be in a state of superposition. This challenges the ability to exactly predict any outcome even if the initial conditions are known. Here is a way to make these concepts more intuitive to grasp. Determinism is Like a Chess Game: All positions are always known with certainty; the effects of any move are also known with certainty; there is no randomness. Furthermore, if every move is known from the start the exact outcome will always be known with certainty. This reflects the exact concepts of determinism. Probabilism is Like a Game of Cards: Before any card is drawn, it exists in a state where all outcomes are possible, each with its own probability. Only until a card is observed do we resolve the uncertainty. This directly mirrors the quantum concepts of superposition and probabilism. To Recap: The concept of superposition in quantum mechanics challenges determinism by introducing probabilism, stating that outcomes have inherent probabilities until observed, at which point one specific outcome is determined.

The last lesson was all about qubits and the potential displayed by them. Now let’s dive into one of the most fascinating and important properties exhibited by qubits: superposition.
What is Superposition?
This is the quantum mechanical principle where a system or particle can exist in multiple states simultaneously until measured. After measurement the particle or system will collapse into one of the states.
How Superposition Challenges Standard Physics: Determinism vs. Probabilism
Determinism is the idea in classical mechanics that every object’s properties can be determined and measured definitively, allowing all outcomes to be predicted if all initial conditions are known.
Probabilism contradicts this line of thinking by stating that events are described by probabilities rather than certainties. This states that each event has a given chance of occurring and state of an object is not known until it is measured. After measurement all the probabilities collapse into one observed outcome. Until this measurement occurs, the outcomes would be in a state of superposition. This challenges the ability to exactly predict any outcome even if the initial conditions are known.
Here is a way to make these concepts more intuitive to grasp.
Determinism is Like a Chess Game:
All positions are always known with certainty; the effects of any move are also known with certainty; there is no randomness. Furthermore, if every move is known from the start the exact outcome will always be known with certainty. This reflects the exact concepts of determinism.
Probabilism is Like a Game of Cards:
Before any card is drawn, it exists in a state where all outcomes are possible, each with its own probability. Only until a card is observed do we resolve the uncertainty. This directly mirrors the quantum concepts of superposition and probabilism.
To Recap:
The concept of superposition in quantum mechanics challenges determinism by introducing probabilism, stating that outcomes have inherent probabilities until observed, at which point one specific outcome is determined.
What's Your Reaction?